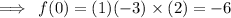Answer:
Degree:3
Y-intercept:-6
Explanation:
The given function is:
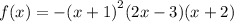
To find the degree, we multiply out the leading terms of each factor.
The leading term of the first factor is:
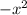
and that of the second factor is
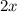
and the third one is:

If we multiply out the leading terms, we would get:
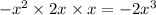
Therefore the degree of the function is:
3
To find the y-intercept, we put x=0 to get: