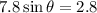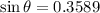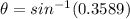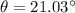Answer:
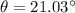
Step-by-step explanation:
Given
velocity of boat with respect to river
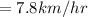
velocity of river is
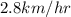
River is 3.5 km wide
Suppose boat leaves at an angle of
 with vertical such that it reaches exactly opposite end of initial Point
with vertical such that it reaches exactly opposite end of initial Point
Therefore
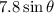 component will balance the river Flowso that sin component helps to cross the river
component will balance the river Flowso that sin component helps to cross the river