Question

You have a bucket full of marbles if the marbles in the bucket are counted by twos threes five and seven there is exactly one left over each time what is the fewest number of marbles that could be in the bucket

Answer 1

There can be a minimum of 211 balls present in the bucket.

Step-by-step explanation:

Given the marbles can be counted by twos, threes, five and seven

Therefore, taking lcm of 2, 3, 5, 7

LCM =
`2* 3* 5* 7 = 210`

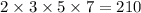
So, 210 balls can be counted in 2’s, 3’s, 5’s and 7’s and it is given that exactly one ball is left after counting

Hence, fewest no. of balls that can be present in the basket = 210+1 = 211

Therefore, there can be a minimum of 211 balls present in the bucket.