Question

Which equation describes this line?

(1.13)
(-2.4)
O A. y-1 = 3(x - 13)
O B. y= 2 = 3(x-4)
O C. y - 4 = 3 (x - 2)
O D . y - 4 = 3(x + 2)

Answer 1

The equation of line with given points is y - 4 = 3 ( x + 2 ) .

Explanation:

Given as :

The points of line are ( 1 , 13 ) and ( - 2 , 4 )

The point slope intercept equation of line is

y -
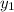`y_1` = m ( x -
`x_1` )

Where m is the slope of line

So , Slope , m =
`(y_2 - y_1)/(x_2 - x_1)`

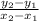
Or, m =
`(4 - 13)/( - 2 - 1)`

Or, m =
`( - 9)/( - 3)`

∴ m = 3

Now The equation of line is

y -
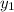`y_1` = m ( x -
`x_1` )

Or. y - 13 = 3 ( x - 1 )

Or, y - 13 = 3 x - 3

Or, y = 3 x - 3 + 13

∴ y = 3 x + 10

I.e y - 4 = 3 x + 6

Or, y - 4 = 3 ( x + 2 )

Hence The equation of line with given points is y - 4 = 3 ( x + 2 ) . Answer