Question

Most geologist believe that the dinosaurs became extinct 65 million years ago when a large comet or asteroid struck the earth, throwing up so much dust that the sun was blocked out for a period of many months. Suppose an asteroid with a diameter of 2.0 km and a mass of 1.0×10^13kg hits the earth with an impact speed of 4.0×10^4m/s. a. What is the earth's recoil speed after such a collision? (Use a reference frame in which the earth was initially at rest.) b. What percentage is this of the earth's speed around the sun? (Use the astronomical data inside the back cover.)

Answer 1

a) v = 6.69 10⁻⁸ m / s, b) 2.3 10⁻¹⁰%

Step-by-step explanation:

This problem is a case of shock, let's use the conservation of the moment, the system is formed by the comet and the Earth. Let's write the moment before and after the crash

The mass of the comet is = 1.0 1013 kg and its velocity vo = 4.0 104 m / s, the mass of the earth is M and its initial velocity is zero (much less than that of the comet)

Initial

p₀ = m v₀

Final

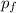`p_(f)` = (m + M) v

As the forces are internal at the moment it is conserved

p₀ =
`p_(f)`

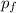
m v₀ = (m + M) v

v = v₀ m / (m + M)

v = 4.0 10⁴ 1.0 10¹³ / (1.0 10¹³ + 5.98 10²⁴)

v = 6.69 10⁻⁸ m / s

b) Let's use the relationship between angular and linear magnitudes

v₁ = w r

w = 2π / T

v₁ = 2πi r / T

Orbit radius = 1,496 10¹¹ m

Period T = 365 days (24 h / 1 day) (3600s / 1h) = 3.15 10⁷ s

v₁ = 2π 1.5 10¹¹ 11 / 3.15 10⁷

v1 = 2.99 10⁴ m / s

% = v/v₁ 100

% = 6.69 10⁻⁸/2.99 10⁴ 100

% = 2.3 10⁻¹⁰%