Question

A consumer upset with the latest trend of postal rate increases has decided to try to send letters by balloon even though they may not reach their intended destinations. A 66400 cm366400 cm3 gas-filled balloon will provide enough lift for a 43.4 g43.4 g package to be accelerated upward at a rate of 3.10 m/s23.10 m/s2 . For these circumstances, calculate the density of the gas the consumer fills the balloon with. The acceleration due to gravity is ????=9.81 m/s2g=9.81 m/s2 and the density of air is ????air=1.16 kg/m3rhoair=1.16 kg/m3 . Neglect the mass of the balloon material and the volume of the package.

Answer 1

d = 0.44 kg/m³

Step-by-step explanation:

To determine the density of the gas into the balloon we need to consider the forces that are acting on the balloon in the x (Fx) and y (Fy) components:

x:
`\Sigma F_(x) = m \cdot a_(x) = 0`

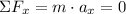
The sum of the forces is zero because there is no force acting on the balloon in this component.

y:
`\Sigma F_(y) = - W_(b) - W_(p) + B = (m_(b) + m_(p)) \cdot a_(y)` (1)

where
`W_(b)` and
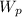`W_(p)`: are the weights of the balloon and the package, respectively,
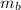`m_(b)` and
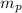`m_(p)`: are the masses of the balloon and the package, respectively, and B: buoyancy force.

Knowing that:

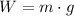`W = m\cdot g` (2)

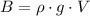`B = \rho \cdot g \cdot V` (3)

where ρ: is the density of the air, g: gravitational acceleration and V: volume of the gas displaced.

And introducing the weights of the balloon and the package from (2), and B from (3) on the equation (1), we can find the mass of the balloon:

`- g (m_(b) + m_(p)) + \rho \cdot g \cdot V = (m_(b) + m_(p)) \cdot a_(y)`

`m_(b) =\frac {\rho \cdot g \cdot V}{(a_(y) + g)} - m_(p)`

`m_(b) = \frac {(1.16 (kg)/(m^(3)))(9.81 \frac {m}{s^(2)})(0.0664 m^(3))}{(9.81 (m)/(s^(2)) + 3.10 \frac {m}{s^(2)})} - 43.4 \cdot 10^(-3)Kg`

`m_(b) = 0.015 kg`

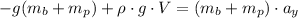
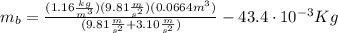
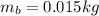
Finally, with the mass of the balloon calculated we can determine the density (d) of the gas inside the balloon:

`d = \frac {m_(b)}{V} = \frac {0.015 kg}{0.0664 m^(3)} = 0.23 (kg)/(m^(3))`

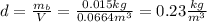
Have a nice day!