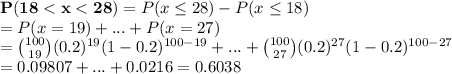Answer:
a) 0.2611
b) 0.6038
Explanation:
We are given the following information:
Let x be a binomial random variable with n = 100 and p = 0.2.
P(Success) = 0.2
Formula:
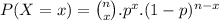
where n is the total number of observations, x is the number of success, p is the probability of success.
a) Now, we are given n = 100 and x = 22
We have to evaluate:
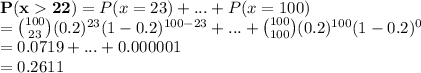
b) Now, we are given n = 100 and 18 < x < 28
We have to evaluate: