Question

A rotating space station is said to create "artificial gravity"—a loosely-defined term used for an acceleration that would be crudely similar to gravity. The outer wall of the rotating space station would become a floor for the astronauts, and centripetal acceleration supplied by the floor would allow astronauts to exercise and maintain muscle and bone strength more naturally than in non-rotating space environments. If the space station is 252 m in diameter, what angular velocity (in rad/s) would produce an "artificial gravity" of 9.80 m/s2 at the rim?

Answer 1

0.278 rad/s

Step-by-step explanation:

d = Diameter of space station = 252 m

r = Radius =
`(d)/(2)=(252)/(2)\ m`

g = Acceleration due to gravity = 9.80 m/s²

`\omega` = angular velocity

Here the centripetal acceleration and the acceleration due to gravity will balance each other

Centripetal acceleration is given by

`a_c=r\omega^2`

`a_c=g\\\Rightarrow r\omega^2=g\\\Rightarrow (252)/(2)\omega^2=9.8\\\Rightarrow \omega=\sqrt{(9.8* 2)/(252)}\\\Rightarrow \omega=0.278\ rad/s`

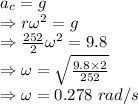
The angular velocity required would be 0.278 rad/s