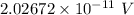Answer:
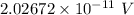
Step-by-step explanation:
f = Frequency = 75 Hz
B = Magnetic field =
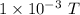
d = Diameter of cell =
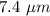
t = Time taken
 = Angular frequency
= Angular frequency
The expression of emf is
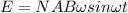
Emf is maximum when
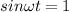 and N = number of turns = 1
and N = number of turns = 1

The maximum emf that can be generated around the perimeter of a cell in this field is