Question

Please help me and tell me how to do it

Answer 1

1520

Explanation:

Thiis is an arithmetic series. We can find "a", "d", and "l" from the series given.

1 + 4 + 7 +...+ 91 + 94

Here,

a is the first term (which is 1)

d is the common difference (the difference in a term and its previous term)

4 - 1 = 3

7 - 4 = 3

and so on...

d = 3

and

l is the last term (which is 94)

Now, from the series given, we can see that there are (32) terms. So

n = 32

Note: 94 - 1 = 93/3 = 31 + 1 = 32

Now, the formula to find the sum of n terms is:

`s_n=(n)/(2)(a+l)`

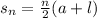
Substituting the values, we find the sum:

`s_n=(n)/(2)(a+l)\\s_(32)=(32)/(2)(1+94)\\s_(32)=16(95)\\s_(32)=1520`

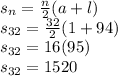
The sum is 1520