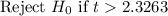Question

The owner of a local nightclub has recently surveyed a random sample of n = 250 customers of the club. She would now like to determine whether or not the mean age of her customers is greater than 30. If so, she plans to alter the entertainment to appeal to an older crowd. If not, no entertainment changes will be made. If she wants to have a level of significance at 0.01, what rejection region should she use?

1. Reject H0 if t < -2.3263.
2. Reject H0 if t > 2.3263.
3. Reject H0 if t < -2.5758.
4. Reject H0 if t > 2.5758.

Answer 1

Option 2.
`\text{Reject}~ H_0~ \text{if}~ t > 2.3263`

Explanation:

We are given the following in the question:

Population mean, μ = 30

Sample size, n = 250

Alpha, α = 0.01

First, we design the null and the alternate hypothesis

`H_(0): \mu = 30\\H_A: \mu > 30`

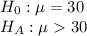
We use Right-tailed t test to perform this hypothesis.

Formula:

`t_(stat) = \displaystyle\frac{\bar{x} - \mu}{(\sigma)/(√(n)) }`

Now,
`t_(critical) \text{ at 0.01 level of significance, 249 degree of freedom } = 2.3263`

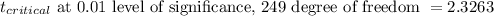
Decision rule:

For a right ailed t-test,

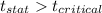`t_(stat) > t_(critical)`, we reject the null hypothesis as it lies in the rejection area.

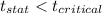`t_(stat) < t_(critical)`, we fail to reject the null hypothesis as it lies in the acceptance area and accept the null hypothesis.

Thus,

Option 2.
`\text{Reject}~ H_0~ \text{if}~ t > 2.3263`