Answer:
Each leg =
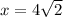
Explanation:
Angle C is the right angle, so the side oppsoite of the right angle is the "HYPOTENUSE", which is 8.
THe legs are AC and BC, which are x each.
Using pythagorean theorem, we can solve for x (length of each leg).
Pythagorean theorem = Leg^2 + Leg^2 = Hypotenuse^2
So we can write:
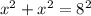
Simplifying and solving for x:
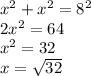
We need to keep an exact answer (with radicals), so we need to simplify this using the two rules of radicals below:

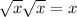
Now, lets solve for x:

This is the exact answer. Each leg length.