Question

A pump is driven by a 2-kW motor with water at 150 kPa, 10 Cesius entering. Find the maximum water flow rate you can get with an exit pressure of 1 MPa and negligible kinetic energies. The exit flow goes through a small hole in a spray nozzle out to the atmosphere at 100 kPa. Find the spray velocity.

Answer 1

42.4m/s

Step-by-step explanation:

To develop the problem it is necessary to apply the concepts related to thermodynamic work and Bernoulli's principle in which the behavior is described of a liquid moving along a stream.

The work of an incomprehensible liquid is given by the equation,

`\dot{W} = \dot{m}w = -\dot{m}\upsilon (P_e-P_i)`

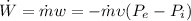
Where,

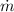`\dot{m}`= Mass flow

`\upsilon` = Specific Volumen

P = Pressure

Our values are given by,

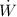`\dot{W}`= 2kW

`P_i = 150kPa\\P_f = 1000kPa\\P_e = 100kPa`

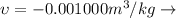`\upsilon = -0.001000 m^3/kg \rightarrow` Table 1 for saturated water in 10°C

We need to find the mass flow, then re-arrange for
`\dot{m}`

`\dot{m} = \frac{\dot{W}}{-\upsilon(P_f-P_i)}`

`\dot{m} = (-2)/(-0.001000(1000-150))`

`\dot{m} = 2.356kg/s`

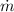
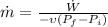
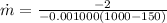
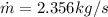
To find the Spray velocity, we apply Bernoulli equation, because at the Nozzle there is not Work or Heat transfer related. Then,

`(1)/(2)V^2 = \upsilon \Delta P`

`V = √(2*\upsilon(P_e-P_i))`

`V = √(2*0.001003(1000-100))`

`V = 42.4m/s`

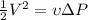
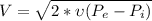
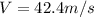
Therefore the spray velocity is 42.4m/s