Question

At some time, a charged particle with q = −1.24×10−8C is moving with instantaneous velocity v⃗ = (4.19×104m/s) i^ + (−3.85×104m/s) j^ . Part A The particle is in a magnetic field B⃗ = (1.50 T ) i^. What is the force on the particle? Enter the x, y, and z components of the force separated by commas, so your answer should be three numbers.

Answer 1

F = (0 i ^ + 0 j ^ -7.16 10⁻⁴ k ^) N

Step-by-step explanation:

The magnetic force is given by the equation

F = q v x B

Where the bold indicate vectors.

One of the easiest ways to solve this vector product with the use of determinants

`F= q \left[\begin{array}{ccc}i&j&k\\4.19&-3.85&0\\1.5&0&0\end{array}\right]` 10⁻⁴

F = -1.24 10-8 10 4 (i ^ (0) + j ^ (0) - k ^ (1.5 (-3.85))

F = -7.16 10⁻⁴ k^ N

The result is given in the way

F = (0 i ^ + 0j ^ - 7.16 10⁻⁴ k^) N

Another way to solve the vector product is to calculate the magnitude

F = q v B sin θ

-If the two vectors are parallel, the vector product is zero.

-If the two vectors are perpendicular, the product is maximum

-The direction is given by the rule of the right hand, the fingers of the hand rotate the first vector over the second or and the thumb points in the direction of the resultant.

Let's apply the above to our case

Speed ​​has components in the x and y direction

The field has component in the x direction, with unit vector (i ^)

From the relationships above

i^ x i^ = 0

i^ x j^ = k ^

j^ x i^ = -k ^

let's calculate the strength

Fx = 0

Fy = 0

Fz = q (vₓ
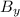`B_(y)`) sin 180

Fz = -1.24 10⁻⁸ (-385 10⁴) 1.5 (-1)

Fz = -7.16 10⁻⁴

It's resulting vector is

F = (0 i ^ + 0 j ^ -7.16 10⁻⁴ k ^) N