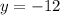For this case we have the following equation:

We apply distributive property to the terms within parentheses:

We subtract
 and on both sides of the equation:
and on both sides of the equation:
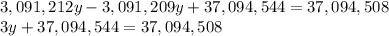
We subtract
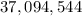 on both sides of the equation:
on both sides of the equation:
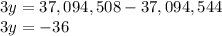
We divide by 3 on both sides of the equation:
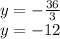
Answer: