Answer:
d) 65.9 in < μ < 67.1 in.
Explanation:
Given that a 99% confidence interval (in inches) for the mean height of a population is 65.7 < μ < 67.3
Mean = average of 65.7 and 67.3 =
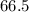
i.e. here margin of error =
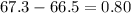
For 99% confidence interval critical value is 2.58
Hence std deviation =

For 95% confidence interval critical value is 1.96
Margin of error =

Hence confidence interval =
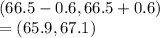
d) 65.9 in < μ < 67.1 in. is right