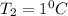Answer:1

Step-by-step explanation:
Let the heat given by flame be
 .
.
Let
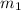 be the mass of water in case 1.
be the mass of water in case 1.
Let
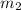 be the mass of water in case 2.
be the mass of water in case 2.
Let Δ
 be the temperature difference in case 1.
be the temperature difference in case 1.
Let Δ
 be the temperature difference in case 2.
be the temperature difference in case 2.
Let
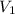 be the volume of water in case 1.
be the volume of water in case 1.
Let
 be the volume of water in case 2.
be the volume of water in case 2.
Let
 be the density of water.
be the density of water.
Let
 be the specific heat of water.
be the specific heat of water.
Given,
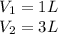
Δ
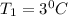
Since the same heat is given to water in both the cases,
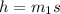 Δ
Δ

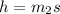 Δ
Δ

So,
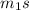 Δ
Δ
 =
=
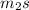 Δ
Δ

Since mass is product of volume an density,
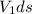 Δ
Δ
 =
=
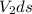 Δ
Δ

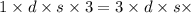 Δ
Δ

So,Δ