Question

It is possible to determine the ionization energy for hydrogen using the Bohr equation. Calculate the ionization energy (in kJ) for a mole of hydrogen atoms, making the assumption that ionization is the transition from

Answer 1

The ionization energy for a mole of hydrogen atoms is 1,312.17 kiloJoules.

Step-by-step explanation:

Energy of the nth orbit by Bohr was given by:

`E_n=-13.6* (Z^2)/(n^2)eV`

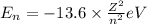
where,

`E_n` = energy of
`n^(th)` orbit

n = number of orbit

Z = atomic number

Energy of the first shell(n=1) in hydrogen atom:

Z = 1

`E_1=-13.6* (1^2)/(1^2)eV=-13.6 V`

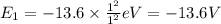
Energy of the first shell(n=∞) in hydrogen atom:

Z = 1

`E_(\infty )=-13.6* (1^2)/((\infty ^2))eV=0`

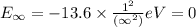
Ionization energy of hydrogen atom,I.E : 1 → ∞

`I.E=E_(\infty )-E_1=0-(-13.6 eV)=13.6 eV`

`1 eV = 1.60218* 10^(-19) J`

`13.6 eV=13.6* 1.60218* 10^(-19) J=2.179* 10^(-18) J`

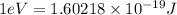
`2.179* 10^(-18) J=2.179* 10^(-21) kJ` (1kJ = 1000J)

Ionization energy of 1 mol = E

1 mol =
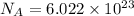`N_A=6.022* 10^(23)` atoms

`E= 6.022* 10^(23)* 2.179* 10^(-21) kJ=1,312.17 kJ`

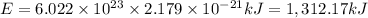
The ionization energy for a mole of hydrogen atoms is 1,312.17 kiloJoules.