Question

Suppose you purchase a bundle of 18 bare-root broccoli plants. The sales clerk tells you that on average you can expect 8% of the plants to die before producing any broccoli. Assume that the bundle is a random sample of plants. Use the binomial formula to find the probability that you will lose at most 1 of the broccoli plants.

Answer 1

There is a 57.18% probability that you will lose at most 1 of the broccoli plants.

Explanation:

For each plant, there are only two possible outcomes. Either they die, or they do not. This means that we can solve this problem using the binomial probability distribution.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

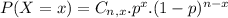
In which
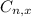`C_(n,x)` is the number of different combinatios of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

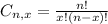
And p is the probability of X happening.

In this problem, we have that

There are 18 plants, so
`n = 18`.

8% of the plants die before producing any broccoli. So
`p = 0.08`.

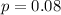Use the binomial formula to find the probability that you will lose at most 1 of the broccoli plants.

This is

`P = P(X = 0) + P(X = 1)`

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

`P(X = 0) = C_(18,0).(0.08)^(0).(0.92)^(18) = 0.2229`

`P(X = 1) = C_(18,1).(0.08)^(1).(0.92)^(17) = 0.3489`

`P = P(X = 0) + P(X = 1) = 0.2229 + 0.3489 = 0.5718`

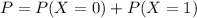
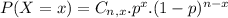
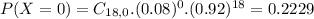
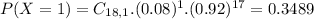
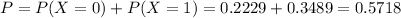
There is a 57.18% probability that you will lose at most 1 of the broccoli plants.