Question

A rectangular football field is

64
6464 meters wide and
100
100100 meters long. A player runs from one corner of the field in a diagonal line to the opposite corner.

Answer 1

118.73 meters (rounded to 2 decimal places)

Explanation:

The question is to find the length (in meters) the player runs.

This is basically the length (in m) of the diagonal of the rectangle.

If we let the length of diagonal be "d", we can use the formula shown below to calculate d:

`d^2=l^2+w^2`

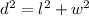
Where

d is the diagonal (what we want to find)

l is the length (given as 100)

w is the width (given as 64)

We substitute and solve for d:

`d^2=l^2+w^2\\d^2=(100)^2 + (64)^2\\d^2 = 14096\\d=√(14096) \\d=118.73`

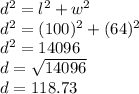
So, the player ran around 118.73 meters