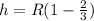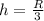Question

A block is balanced on top of a frictionless sphere of radius R. When the block is given a slight nudge it starts to slide down the surface of the sphere. How far (measured vertically) below the top of the sphere does the block lose contact with the surface of the sphere?

Answer 1

Answer:
`(R)/(3)`

Step-by-step explanation:

Given

Sphere of Radius R

Suppose mass of block is m

At any instant \theta Normal reaction(N) and weight(mg) is acting such that

`mg\sin \theta -N=(mv^2)/(R)` , where v is velocity of block at any angle \theta

When block is just about to leave then N=0

therefore

`mg\sin \theta =(mv^2)/(R)`

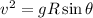`v^2=gR\sin \theta`-------------------1

Also by conserving Energy we get

Potential Energy=kinetic Energy of block

`mgh=(mv^2)/(2)`

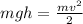
here h=vertical distance traveled by block

From diagram

`h=R-R\sin \theta`

`h=R(1-\sin \theta )`

`mgR(1-\sin \theta )=(mv^2)/(2)`

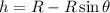
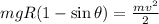
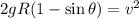`2gR(1-\sin \theta )=v^2`-----------------2

From 1 and 2

`2(1-\sin \theta )=\sin \theta`

`3\sin \theta =2`

`\sin \theta =(2)/(3)`

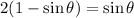
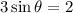
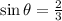
Thus from this value of h is

`h=R(1-\sin \theta )`

`h=R(1-(2)/(3))`

`h=(R)/(3)`