Answer: -3.
Explanation:
We are given that :
 (1)
(1)
It means that f(x) and g(x) are proportional.
[Equation of direct variation: y=kx , where k= proportionality constant.]
From the given graph ,
At x= -3 , f(x)=1 and g(x) =-3
Put value of x=-3 in (1) , we get
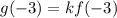
 [∵ f(x)=1 and g(x) =-3]
[∵ f(x)=1 and g(x) =-3]
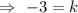
i.e. The value of k = -3
Hence, the correct answer is -3.