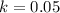Question

The equation 5y = 0.25x represents a proportional relationship. What is the constant of proportionality?

Answer 1

The Constant of proportionality is:

`k=0.05`

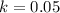
Step-by-step explanation:

To solve this exercise it is important to remember that, by definition, a Proportional relationship has the following form:

`y=kx`

Where "k" is the Constant of proportionality.

In this case the exercise gives you the following equation that represents a Proportional relationship:

`5y = 0.25x`

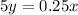
In order to find the Constant of Proportionality, you must solve for "y".

To solve for "y" you can divide both sides of the equation by 5. Then:

`(5y)/(5)=(0.25x)/(5)\\\\y=0.05x`

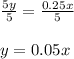
Therefore, you can identify that the Constant of Proportionality is:

`k=0.05`