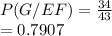Answer:
Explanation:
Given that a box contains 45 light bulbs, of which 36 are good and the other 9 are defective.
Probability for any one to be defective as of now is
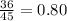
a)
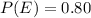
b) After I draw we have 35 good ones and 9 defective
P(F/E) =

c) P(G/EF)
This is the probability for iii bulb to be good given I and II are goog.
Once I and ii are good we have 43 bulbs with 34 good ones