Question

Select the correct answer from each drop-down menu.

A perpendicular bisector, , is drawn through point C on .
If the coordinates of point A are (-3, 2) and the coordinates of point B are (7, 6), the x-intercept of is . Point lies on .

Answer 1

The following steps are needed:

1. Find the function of AB with A(-3,2) and B(7,6)

y(AB) = mx + b. Calculate m = (y₂-y₁)/(x₂-x₁) = (6-2)/(7+3) = 4/10 → 2/5

y(AB) = (2/5).x + b. Calculate b; 6 = (2/5).(7) + b and b = 16/5

y(AB) = (2/5).x +16/5

2. calculate the function y(CD) = mx + bWe know already m = -5/2, since CD is perpendicular to AB and hence the product of their slopes = -1

y(CD) = -(5/2).x + b

We also know that y(CD) passes in the middle of AB, then let's calculate the coordinate of C, the midpoint of AB:x=(x₁+x₂)/2 and y=(y₁+y₂)/2)x= (-3+7)/2 and y=(2+6)/2x= 2 and y=4y(CD) = (-5/2).x +b4 = (-5/2).(2)+b and b = 9y(CD) =(-5/2)x + 9

x intercept when y = 0 → 0= (-5/2)x +9 → x= 18/5So x intercept (18/5,0)

So the answer is at point B.

Answer 2

The x- intercept of CD is B(18/5,0) and point C(32,-71) lies on CD

Explanation:

We are given that a perpendicular bisector CD is drawn through point C on AB.

The coordinates of point A are (-3,2)

The coordinates pf point B are (7,6).

We have to find the x-intercept of CD

Slope formula:
`m=(y_2-y_1)/(x_2-x_1)`

By using the formula

Slope of AB=
`(6-2)/(7+3)=(4)/(10)=(2)/(5)`

Slope of CD=
`-(1)/((2)/(5))=-(5)/(2)`

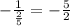
When two lines are perpendicular then slope of one line is equal to negative reciprocal of slope of other line.

Mid point of AB

`x=(-3+7)/(2)=2,y=(2+6)/(2)=4`

The mid-point of AB is at (2,4)

By using mid-point formula

`x=(x_1+x_2)/(2),y=(y_1+y_2)/(2)`

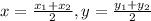
The equation of line CD is passing through the point (2,4) with slope -5/2 is given by

`y-4=-(5)/(2)(x-2)`

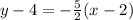
By using point slope form:
`y-y_1=m(x-x_1)`

`y=-(5)/(2)(x-2)+4`

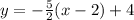
Substitute y=0

`-4=-(5)/(2)(x-2)`

`x-2=(4* 2)/(5)`

`x-2=(8)/(5)`

`x=(8)/(5)+2=(8+10)/(5)`

`x=(18)/(5)`

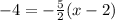
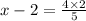
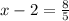
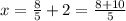
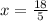
Hence, the x- intercept of CD is (18/5,0).

`y=-(5)/(2)(x-2)+4`

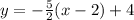
Substitute x=-52

`y=-(5)/(2)(-52-2)+4=139`

Substitute x=-20

`y=-(5)/(2)(-20-2)+4=59`

Substitute x=32

`y=-(5)/(2)(32-2)+4=-71`

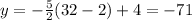
Substitute x=-54

`y=-(5)/(2)(-54-2)+4=144`

Hence, point C(32,-71) lies on CD.