Question

A consumer believes that a certain potato chip maker is putting fewer chips in their regular bags of chips than the advertised amount of 12 ounces. In order to test the null hypothesis that the average chip weight is 12 ounces per bag vs. the alternative hypothesis that the average chip weight is less than 12 ounces per bag, a random sample of 49 bags were selected. The resulting data produced a p - value of 0.136.(a) At a 5% level of significance, should the null hypothesis be rejected? (Type: Yes or No):(b) At a 10% level of significance, should the null hypothesis be rejected? (Type: Yes or No): In a statistical test of hypotheses, saying that ''the evidence is statistically significant at the .05 level'' meansA. the p - value is at least .05.B. the p - value is less than .05.C. ? is more than .25.D. ?=.10.

Answer 1

a) No

b) No

c) P value is more than 0.05.

Explanation:

We are given the following in the question:

Population mean, μ = 12 ounces

Sample size, n = 49

P-value = 0.136

First, we design the null and the alternate hypothesis

`H_(0): \mu = 12\text{ ounces}\\H_A: \mu < 12\text{ ounces}`

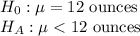
We use One-tailed z test to perform this hypothesis.

a) Alpha, α = 0.05

Since, p-value > α,

The null hypothesis should not be rejected. We accept the null hypothesis and reject the alternate hypothesis. We conclude that the average chip weight is 12 ounces per bag.

b) Alpha, α = 0.10

Since, p-value > α,

The null hypothesis should not be rejected. We accept the null hypothesis and reject the alternate hypothesis. We conclude that the average chip weight is 12 ounces per bag.

c) The evidence is statistically significant at the .05 level means that the p value is more than 0.05.