Question

How many chocolates are in the 2nd jar ?

Answer 1

12

Explanation:

Let x be the number of chocolates in the first jar, then

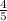`(4)/(5)` x ← number of chocolates in second jar and

x + 90 ← number of chocolates in the third jar

The second jar also contains one half the chocolates in the third jar, thus

Equating chocolates in second jar

`(4)/(5)` x =
`(1)/(2)`(x + 90) =
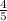`(1)/(2)` x + 45

Multiply both sides by 10 to clear the fractions

8x = 5x + 450 ( subtract 5x from both sides )

3x = 450 ( divide both sides by 3 )

x = 150

and
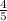`(4)/(5)` × 150 = 120

Number of chocolates in second jar = 120