The length of side of triangle is 5 units and length of side of pentagon is 1.5 units.
SOLUTION:
Given, An equilateral triangle has sides of length
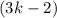 units.
units.
Then, perimeter of the triangle will be

A regular pentagon (5 sides) has sides of
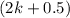 units.
units.
Then, perimeter of the pentagon will be
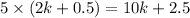
We have to find the dimensions of each shape . Now, the perimeter of the triangle is twice the perimeter of the pentagon,
So,

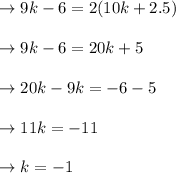
Then, length of side of triangle
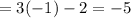
Length of side of pentagon
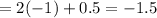
We have to neglect, negative sign as lengths can’t be negative. Even if we change the sign above all conditions are satisfied.