Question

What is the distance between point A -1,3 and point B -7,3

Answer 1

the distance is -6

Explanation:

a to 0,0 is 1

b to 0,0 is 7

1-7= -6

Answer 2

The distance between point A (-1, 3) and point B (-7, 3) is 6 units

Solution:

Given, two points are A(-1, 3) and (-7, 3)

We need to find the distance between above two points.

We know that distance between two points
`P\left(x_(1), y_(1)\right) \text { and } Q\left(x_(2), y_(2)\right)` is given as:

`d(P, Q)=\sqrt{\left(x_(2)-x_(1)\right)^(2)+\left(y_(2)-y_(1)\right)^(2)}`

`\text { here, } x_(1)=-1, y_(1)=3, x_(2)=-7 \text { and } y_(2)=3`

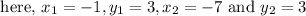
Substitute the values we get,

`\begin{array}{l}{\text { Distance }=\sqrt{((-7)-(-1))^(2)+(3-3)^(2)}} \\\\ {=\sqrt{(1-7)^(2)+(0)^(2)}=\sqrt{(-6)^(2)+0}} \\\\ {=√(36)=6}\end{array}`

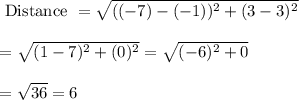
Hence, the distance between two points is 6 units.