Question

Calculate the Gibbs energy of freezing (DeltaGfreezing) in units of J/mol when supercooled water freezes at -3degreeC at constant T and P. Delta H fusion = 6000 J/mol at 0degreeC. The molar heat capacity of water and ice are 75.3 J/molK and 38 J/molK, respectively, and both are independent of temperature over this range. State any assumptions you make in your calculation!

Answer 1

Step-by-step explanation:

It is known that the change in Gibb's free energy varies with temperature as follows.

`\Delta G(T) = \Delta H(T) - T \Delta S(T)`

=
`\Delta H(T_(f)) - \Delta C_(p,m) (T - T_(f)) - T[\Delta S(T_(f)) - \Delta C_(p,m) ln ((T)/(T_(f)))]`

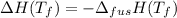`\Delta H(T_(f)) = -\Delta_(fus) H(T_(f))` (assumption)

=
`\Delta H(T_(f)) - (T)/(T_(f)) \Delta H(T_(f)) - \Delta C_(p, m)(T - T_(f) - T ln (T)/(T_(f)))`

=
`((T)/(T_(f)) - 1) \Delta_(fus) H(T_(f)) - \Delta C_(p,m)(T - T_(f) - Tln ((T)/(T_(f))))`

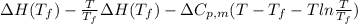
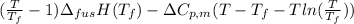
As, T =
`-3^(o)C` = (-3 + 273) = 270 K,
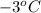`T_(f) = 0^(o)C = 0 + 273 K = 273 K`.

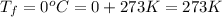Therefore, calculate the change in Gibb's free energy as follows.

`\Delta G(T) = ((T)/(T_(f)) - 1) \Delta_(fus) H(T_(f)) - \Delta C_(p,m)(T - T_(f) - Tln ((T)/(T_(f))))`

=
`((270 K)/(273 K) - 1)(6000 J/mol K) - (75.3 - 38) J/mol K (270 K - 273 K - 270 K ln (270 K)/(273 K))`

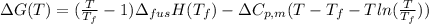
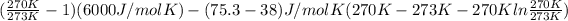
= -65.93 J/mol K + 0.62 J/mol K

= -65.31 J/mol K

Thus, we can conclude that Gibbs energy of freezing for the given reaction is -65.31 J/mol K.