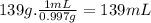Question

How many milliliters of water at 25.0°C with a density of 0.997 g/mL must be mixed with 162 mL of coffee at 94.6°C so that the resulting combination will have a temperature of 62.5°C? Assume that coffee and water have the same density and the same specific heat (4.18 J/g·°C) across the temperature range.

Answer 1

The volume of water is 139 mL.

Step-by-step explanation:

Due to the Law of conservation of energy, the heat lost by coffee is equal to the heat gained by the water, that is, the sum of heats is equal to zero.

`Q_(coffee) + Q_(water) = 0\\Q_(coffee) = - Q_(water)`

The heat (Q) can be calculated using the following expression:

`Q=c * m * \Delta T`

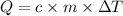
where,

c is the specific heat of each substance

m is the mass of each substance

ΔT is the difference in temperature for each substance

The mass of coffee is:

`162mL.(0.997g)/(mL) = 162g`

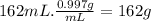
Then,

`Q_(coffee) = - Q_(water)\\c_(c) * m_(c) * \Delta T_(c) = -c_(w) * m_(w) * \Delta T_(w)\\m_(c) * \Delta T_(c) = -m_(w) * \Delta T_(w)\\m_(w) = (m_(c) * \Delta T_(c))/(-\Delta T_(w)) \\m_(w)=(162g * (62.5 \°C - 94.6 \°C ) )/(-(62.5 \°C - 25.0 \°C)) \\m_(w) = 139 g`

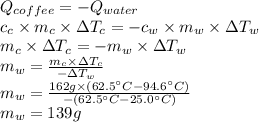
The volume of water is:

`139g.(1mL)/(0.997g) =139mL`