Question

The leg of a right triangle is 3 units and the hypotenuse is 6 units. What is the length, in units, of the other leg of the triangle?

Answer 1

about 5.2

Explanation:

a^2+b^2=c^2

Answer 2

The length, in units, of the other leg of the triangle is
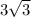`3√(3)` units

Solution:

Given that, The leg of a right triangle is 3 units and the hypotenuse is 6 units.

We have to find what is the length, in units, of the other leg of the triangle

For any right angled triangle, by Pythagorean theorem, square of the length of the hypotenuse is equal to the sum of squares of the lengths of other two sides of the right-angled triangle.

`c^(2)=a^(2)+b^(2)`

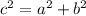
Where "c" is the length of hypotenuse

"a" is the length of one side of right angled triangle

"b" is the length of other side of right angled triangle

Substituting the values we get,

`6^(2)=3^(2)+b^(2)`

`\begin{array}{l}{\rightarrow 36=9+b^(2)} \\\\ {\Rightarrow b^(2)=36-9} \\\\ {\Rightarrow b^(2)=27} \\\\ {\Rightarrow b=√(27)} \\\\ {\Rightarrow b=3 √(3)}\end{array}`

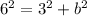
Hence, the length of the other leg is
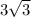`3√(3)` units