Question

The size of a computer monitor is usually given by length of its diagonal.A monitor's aspect ratio is the ratio of its width to its height .This monitor has a diagonal length of 19 inches and an aspect ratio of 5:4 .What are the width and height of the monitor? Round to the nearest tenth of an inch

Answer 1

The width of the monitor is approximately 9.7 inches and the height is approximately 7.8 inches.

Step-by-step explanation:

To find the width and height of the monitor, we can use the Pythagorean theorem. Let's assume the width is 5x and the height is 4x. The diagonal is 19 inches, so we can set up the equation: (5x)^2 + (4x)^2 = 19^2. Simplifying, we get 41x^2 = 361. Dividing both sides by 41, we find x^2 = 361/41. Taking the square root, we get x = √(361/41). So, the width is approximately 9.7 inches (5 * √(361/41)) and the height is approximately 7.8 inches (4 * √(361/41)). Rounding to the nearest tenth, the width is 9.7 inches and the height is 7.8 inches.

Answer 2

The Length of monitor is 14.84 inches And Width of monitor is 11.87

Step-by-step explanation:

Given as :

The length of diagonal of rectangular monitor = 19 inches

The length to width ratio is 5:4

So , Let the Length of monitor = 5x

and The Width of monitor = 4x

Now, From Pythagoras theorem

(Diagonal)² = Length² + width²

Or, 19² = (5x)² + (4x)²

Or, 361 = 25x² + 16x²

Or, 41x² = 361

So, x² = 8.804

∴ x =
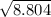`√(8.804)` = 2.967 inches

So, The length = 5x = 14.835 inches

And The width = 4x = 11.868 inches

Hence The Length of monitor is 14.84 inches And Width of monitor is 11.87 Answer