Answer:
There is a 0.58% probability of Florida being struck by four or more hurricanes in the same year.
Explanation:
Since we have only the mean during the interval, we can solve this problem using the Poisson probability distribution.
In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:
Poisson probability distribution
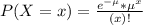
In which
x is the number of sucesses
 is the Euler number
is the Euler number
 is the mean in the given time interval.
is the mean in the given time interval.
In this problem, we have that:
Since 1851, exactly 119 hurricanes have hit Florida (this includes the years 1851 and 2019). Counting 1851 and 2019, there are 169 years in this interval. This means that
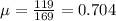
If the probability of hurricane strikes has remained the same since 1851, what is the probability of Florida being struck by four or more hurricanes in the same year?
This is
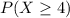 .
.
Either Florida is struck by less than four hurricanes in a given year, or it is struck by 4 or more. The sum of these probabilities is decimal 1.
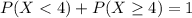
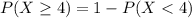
In which
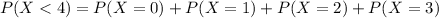
So
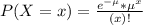
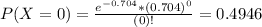
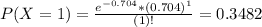
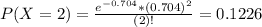
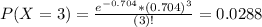

Finally
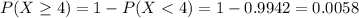
There is a 0.58% probability of Florida being struck by four or more hurricanes in the same year.