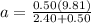Answer:
Part a)

Part b)
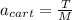
Part c)
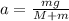
Part d)

Step-by-step explanation:
Part a)
For hanger we know that it will have tension force upwards while it has downwards its weight so we will have
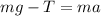
so we have

Part b)
now for car that is rolling on the floor the net force is given as
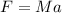
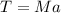
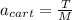
Part c)
now we know that the cart and the hanger both are connected to each other
so they must have same acceleration
so we will have
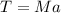
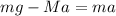
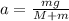
Part d)
now we know that
M = 2.40 kg
m = 0.50 kg
so we will have