Answer:
a) Y = 500
b) Wages: 2.5
Rental price: 2.5
c) labor Share of output: 0.370511713 = 37.05%
Step-by-step explanation:
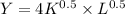
if K = 100 and L = 100
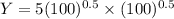
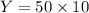
Y = 500
wages: marginal product of labor = value of an extra unit of labor
dY/dL (slope of the income function considering K constant while L variable)
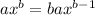

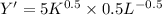
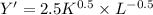
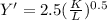
With K = 100 and L = 100
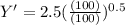
Y' = 2.5
rental: marginal product of land = value of an extra unit of land
dY/dK (slope of the income function considering K variable while L constant)

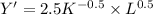
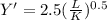
L = 100 K = 100
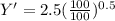
Y' = 2.5
c) we use logarithmic properties:
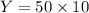
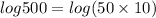
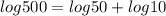
50 was the land while 10 the labor
2.698970004 = 1.698970004 + 1
share of output to labor: 1/2.698970004 = 0.370511713