Answer:252 miles
Step-by-step explanation:
Given
During his way to mountain it took 7 hr to drive
and during his return trip it took 4 hr to return
Let x be the distance between home and mountain
average speed for return is 27 miles per hour faster than his former trip
let v be the speed on his way to mountain thus v+27 is his return speed
thus
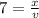 ----1
----1
for return trip
 -----2
-----2
divide 1 & 2


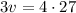
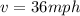
thus
