 will not have real roots that means quadratic equation do not have real solution.
will not have real roots that means quadratic equation do not have real solution.
Solution:
Need to determine the real solutions for following quadratic equations

First let’s check whether the given quadratic equation have real roots or not
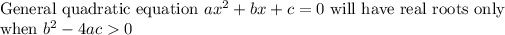
In our case, equation is

Here a = -4, b = 5 and c = -4
Substituting the values in
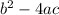
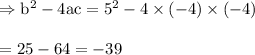
So since in our case
 which is not greater than zero, so quadratic equation
which is not greater than zero, so quadratic equation
 will not have real roots that means quadratic equation do not have real solution.
will not have real roots that means quadratic equation do not have real solution.