Answer:
2√5
Explanation:
The formula for the distance between a point (x, y) and a line in general form, ax +by +c = 0 is ...
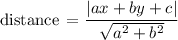
The general form of your equation for the line is ...
2x +y -6 = 0
so the distance to point (x, y) = (4, 8) is ...
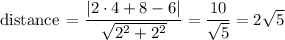
The distance between the point and line is 2√5.