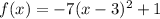Question

Write the equation of the parabola in vertex form.
vertex (3,1), point (2, - 6)
f(x) =?

Answer 1

`f(x)=-7(x-3)^2+1`

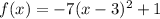
Explanation:

Vertex form of a quadratic is given by:

`f(x)=a(x-h)^2+k`

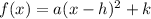
Where (h, k) is the vertex and a is the leading coefficient.

We are given that the vertex is (3, 1). Hence, h = 3 and k = 1. By substitution:

`f(x)=a(x-3)^2+1`

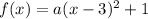
We are also given a point (2, -6). This means that when x = 2, f(x) = -6. Hence:

`-6=a((2)-3)^2+1`

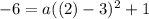
Solve for a. Subtract:

`-6=a(-1)^2+1`

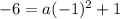
Simplify:

`-6=a+1`

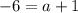
Therefore:

`a=-7`

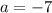
Hence, our quadratic is:

`f(x) = -7 (x-3)^2 + 1`