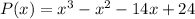Question

What is the polynomial function of least degree whose only zeros are −4 , 2, and 3?

*What do I put in the boxes?

Answer 1

`P(x)=x^3-x^2-14x+24`

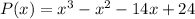
Explanation:

The given zeros are −4 , 2, and 3.

Factor Theorem:

• If a, b and c are the zeros of a polynomial then (x-a) , (x-b) and (x-c) must be the factors. And the polynomial is P(x)=(x-a)(x-b)(x-c)

Using the factor theorem, (x+4), (x-2) and (x-3) are the factors of the polynomial.

Therefore, the polynomial is given by

`P(x)=(x+4)(x-2)(x-3)`

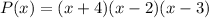
On simplifying, we get

`P(x)=x^3-x^2-14x+24`