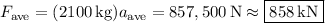Question

IM A 2100-kg pile driver is used to drive a steel I-beam into the ground. The pile driver falls 5.00 m before coming into contact with the top of the beam, and it drives the beam 12.0cm farther into the ground before coming to rest. Using energy considerations, calculate the average force the beam exerts on the pile driver while the pile driver is brought to rest.

Answer 1

The force exerted by the beam imparts an average acceleration with magnitude
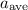`a_(\rm ave)` such that

`0^2 - v^2 = -2a_(\rm ave) (0.12\,\mathrm m) \implies a_(\rm ave) = (v^2)/(0.24\,\rm m)`

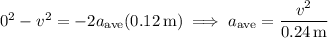
where
`v` is the speed of the pile driver at the moment it first touches the beam.

Presumably, the pile driver is dropped from rest, so any work done on the pile driver as it falls is done exclusively by gravity. Initially, the pile driver has potential energy

`P = (2100\,\mathrm{kg}) g (5.00\,\mathrm m) = 102,900 \,\rm J`

which, assuming no friction or air resistance is involved, gets totally converted to kinetic energy. Then the speed of the pile driver is such that

`K = P \implies 102,900 \,\mathrm J = \frac12(2100\,\mathrm{kg})v^2 \implies v^2 = 98(\rm m^2)/(\rm s^2)`

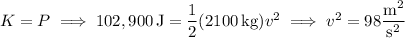
and so the average acceleration of the pile driver as it comes to a stop has magnitude

`a_(\rm ave) = (98(\rm m)/(\rm s))/(0.24\,\rm m) \approx 408(\rm m)/(\rm s^2)`

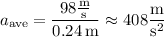
Hence the beam exerts an average force of magnitude

`F_(\rm ave) = (2100\,\mathrm{kg}) a_(\rm ave) = 857,500\,\mathrm N \approx \boxed{858\,\mathrm{kN}}`