Question

(08.04 MC

Find the sum of the geometric sequence 3, 15, 75, 375, ... When there are 8 terms and select the correct answer below.

Answer 1

The sum of next 8 terms is 292,968

Explanation:

Here, the sequence is 3, 15, 75 , ...

Since the given term is in geometric progression, so

`r = (a_2)/(a_1)   = (a_3)/(a_2)`

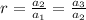
And here, r = 15/ 3 = 5

So, a = 3

r = 5

n = 8

So, sum of n terms of G. P
`S_n= (a(r^(n) -1))/((r-1))`

or,
`S_8= (3(5^(8) -1))/((5-1))`

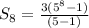
= 292,968

Hence, the sum of next 8 terms is 292,968.