Question

A husband and wife discover that there is a 12​% probability of their passing on a hereditary disease to any of their children. If they plan to have three ​children, what is the probability of the event that at least one child will inherit the​ disease?

Answer 1

Answer: 0.318528

Explanation

Let x be the binomial variable (for success) that represents the children that have a hereditary disease

with parameter p = 0.12 n= 3

Using binomial , we have

`P(x)=^nC_xp^x(1-p)^(n-x)`

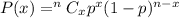
Required probability :-

`P(x\geq1)=1-P(x=0)\\\\=1-^(3)C_(0)(0.12)^(0)(1-0.12)^(3)\\\\=1-(1)(0.88)^(3)}\\\\=0.318528`

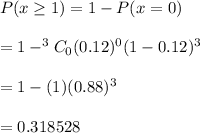
Hence, the probability of the event that at least one child will inherit the​ disease =0.318528