Answer:
"by multiplying each term by 4"
Step-by-step explanation:
Given
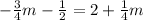
The given equation is in the fraction form.
Now we need to find the least common factor that is the LCM.
Therefore,
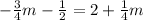
The least common multiple of the above terms is 4
Therefore by multiplying each term of the equation by 4, we get,
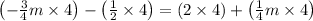
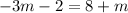
Thus by multiplying each terms by 4 we can eliminate the fraction.
Thus the answer is "by multiplying each term by 4"