Answer:
1.8 mph
Explanation:
first, let's convert 3 hour 40 minutes into hours (to keep same units).
40 minutes is 40/60 = 2/3 hour
So 3 hr 40 minutes =
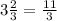 hours
hours
Now, we know D = RT
where D is distance, R is rate, and T is time
Let rate of steamer be x (which is 18)
and rate of current be c
Rate Downstream = x + c (goes with current)
Rate Upstream = x - c (goes against current)
Both Distance (upstream & downstream) are same. Thus, we can write:
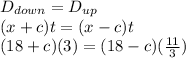
Now, we solve this algebraically for "c", speed of current:
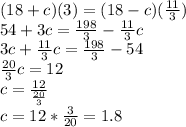
Thus, speed of current = 1.8 mph