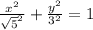Question

4. Use your answer to Problem 3 to find the equation of the ellipse for each of the situations below.

a. An ellipse centered at the origin with x-intercepts (−2,0), (2,0) and yy-intercepts (0,8), (0, −8)
b. An ellipse centered at the origin with x-intercepts (−√5, 0), (√5, 0) and yy-intercepts (0,3), (0, −3)

Answer 1

Explanation:

(a)Ellipse centered at the origin with x-intercepts (−2,0), (2,0) and yy-intercepts (0,8), (0, −8)

as the length of x intercept is smaller than y intercept therefore it is a vertical Ellipse of the form

`(x^2)/(b^2)+(y^2)/(a^2)=1`

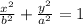
length of major axis
`=2a=2* 8=16`

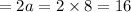
Length of minor axis
`=2b=2times 2=4`

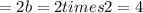
thus Equation of Ellipse is

`(x^2)/(2^2)+(y^2)/(8^2)=1`

(b)x intercept is
`(-√(5),0) (√(5),0)`

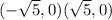
Y intercept is (0,3) (0,-3)

here also Y intercept is greater than x intercept thus it is also a vertical ellipse

length of major axis
`=2a=2* 3=6`

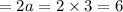
Length of minor axis
`=2b=2* √(5)=2√(5)`

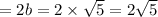
thus Equation of Parabola is

`(x^2)/(√(5)^2)+(y^2)/(3^2)=1`