Question

how many poker hands contain no ace, exactly two queens, and at least one heart? (a poker hand contains five cards.)

Answer 1

Number of hands with 0 aces, 2 non-heart queens, and at least 1 other heart:

`\displaystyle \binom40 \binom 32 \sum_(i=1)^3 \binom{11}i \binom{34}{3-i} = 24618`

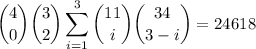
• there are 4 aces to choose from - we want none of them

• there are 3 non-heart queens to choose from - we want exactly 2 of them

• the remaining 3 cards can be 1, 2, or 3 of the 11 available hearts (13 - 1 ace - 1 queen); if
`i` hearts are chosen, then the other
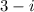`3-i` cards are non-hearts, of which there are 34 cards (52 - 13 hearts - 3 aces - 2 non-heart queens)

Number of hands with 0 aces, 1 heart and 1 non-heart queen, and at least 1 other heart:

`\displaystyle \binom40 \left(\binom11 \binom31\right) \sum_(i=1)^3 \binom{11}i \binom{35}{3-i} = 25905`

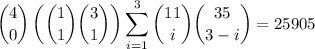
• again, there are 4 aces to choose from, which we don't want

• there is only 1 queen of hearts, and 3 other suits from which to obtain the other queen

• the remaining 3 cards can have
`i\in\{1,2,3\}` hearts from the available 11 hearts (13 - 1 queen - 1 ace), and the other
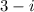`3-i` cards can be chosen from the remaining 35 cards in the deck (52 - 13 hearts - 3 aces - 1 non-heart queen)

Then there is a total of 24618 + 25905 = 50523 such hands.