Answer:
A sample of n = 100 scores with M = 104 gives the most extreme z-score.
Explanation:
The difference between sample mean and population mean is (Standard Error of the Mean) can be written by the formula
SE=(M-m)=
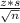 where M is the sample mean, m is the population mean, z is the z-score, s is the population standard deviation, n is the size of the sample. From this we can find out that
where M is the sample mean, m is the population mean, z is the z-score, s is the population standard deviation, n is the size of the sample. From this we can find out that
z=(M-m)×
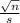

- A sample of n = 25 scores with M = 102 gives
z=(102-100)×
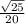 =0.5
=0.5
- A sample of n = 100 scores with M = 102 gives
z=(102-100)×
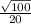 =1
=1
- A sample of n = 25 scores with M = 104 gives
z=(104-100)×
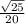 =1
=1
- A sample of n = 100 scores with M = 104 gives
z=(104-100)×
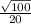 =2
=2