Question

Garden A has 9 more currant bushes than Garden B. If 3 currant bushes are transplanted from Garden B to Garden A, then Garden A will have 1.5 times more currant bushes than Garden B. How many currant bushes are in Garden A before the transplant?

Answer 1

Garden A has 42 currant bushes before the transplant

Explanation:

Suppose garden A is x and garden B is y

Forming the linear equations

Garden A has 9 more currant bushes than garden B

Therefore

x=y+9 --------(1)

If 3 currant bushes are transplanted from Garden B to Garden A, then Garden A will have 1.5 times more currant bushes than Garden B.

When 3 currant bushes are transplanted then the new equation becomes

x=y+12--------(2)

Further after transplanting

x+3= 1.5y----------(3)

putting the value of y from equation 2 into equation 3

x+3 = 1.5(x-12)

x+3= 1.5x-18

x-1.5x=-18-3

-0.5x=-21

`x=(21* 10)/(5)`

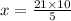
x=42

Hence garden A has 42 currant bushes before the transplant

Answer 2

42

Explanation:

Let x = the original number of bushes in garden A

Let x-9 = the original number of bushes in garden B

x-9-3=x-12 = bushes in garden B after transplanting 3

x+3 = bushes in garden A after transplanting 3

x+3=1.5(x-12)

x+3=1.5x-18

0.5x=21

x=42

There were 42 current bushes in garden A